上 y=x^2 2 parabola 301217-Y=x^2 parabola graph

File Parabola Y X 6x 7 Svg Wikipedia

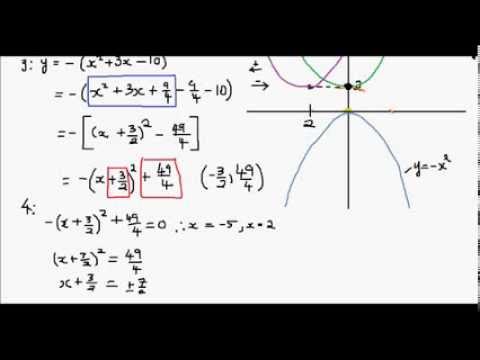
A parabola is the set of points equidistant to a given point (the focus) and straight line (the directrix) The curve mathx=y^2 /math is a parabola with a whose focus is math (\frac {1}The steps are explained with an example where we will find the vertex of the parabola y = 2(x 3) 2 5 Step 1 Compare the equation of the parabola with the vertex form y = a(x h) 2 k and

Y=x^2 parabola graph

Y=x^2 parabola graph-The parabola of quadratic function passes through an only a single point at the yaxis, xintercepts The points at which the parabola graph passes through the xaxis, are called xintercepts, whichThe coordinates that the parabola crosses the y axis can be obtained by solving for y when x=0 That is, y=0²2×015=0015=15 The coordinate of the point for which the parabola crosses

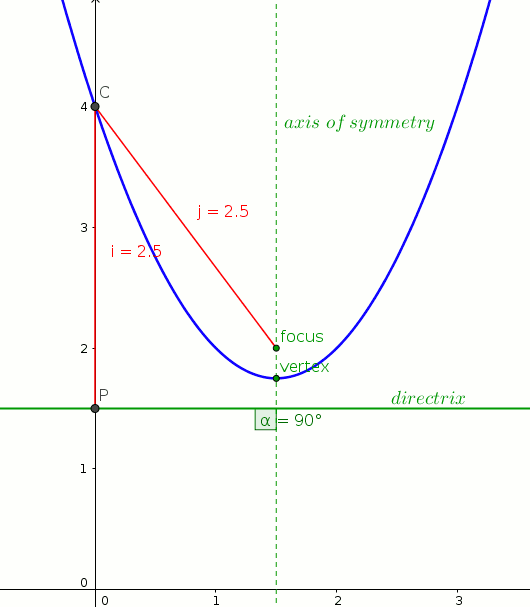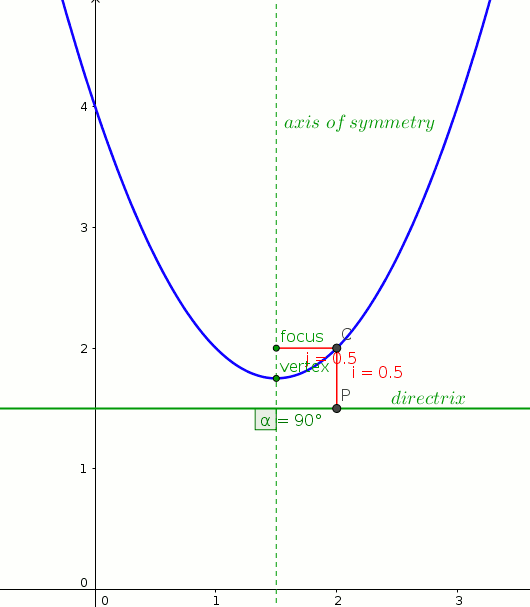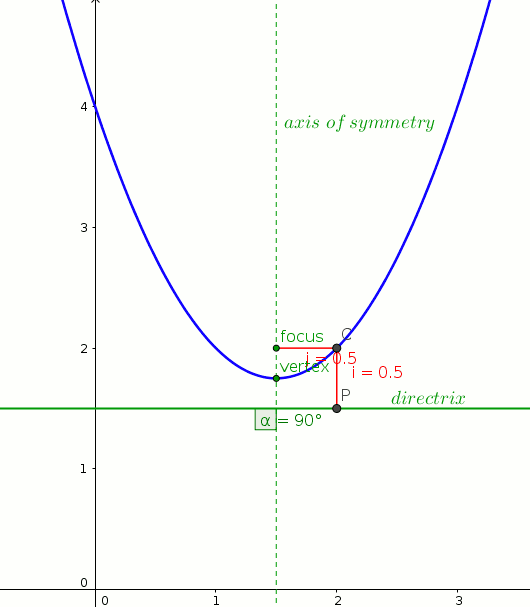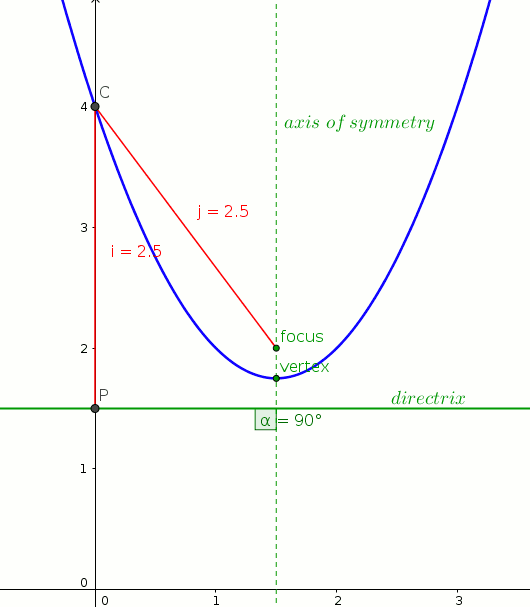
What Are The Vertex Focus And Directrix Of Y X 2 3x 4 Socratic

Y = 2x2 12x 16 A The parabola is y=2x212x16 The vertex of the parabola is, at which point the function We have given that the parabola y=x^2 is shifted up by 2 units and to the right by 3 units Therefore k=3 moves it to the right 3 h=2 moves it up 2 What is the equation of shifting aX = y 2 The vertex of a parabola is the point where the parabola changes direction, and where the graph is most curved On graphs of quadratics, it is found at the very top or bottom of the

 If line y = kx 4 is tangent to parabola y = x x2 at point 'P' then find slope of 'OP' where 'O' is vertex of parabola (1) − 3 2 − 3 2 (2) − 5 4 − 5 4 (3) − 5 2 − 5 2 (4) 3 2 3 2 jee mainConsider the parabola y = x^2 The shaded area is Class 12 >> Maths >> Application of Integrals >> Area Under Simple Curves >> Consider the parabola y = x^2 The shad Question Consider theY = 1 4 p x 2 The diameter and depth given may be interpreted as a point of coordinates ( D / 2, d) = ( 115, 035) on the graph of the parabolic reflector Hence the equation 035 = 1 4 p ( 115) 2

Y=x^2 parabola graphのギャラリー

各画像をクリックすると、ダウンロードまたは拡大表示できます

Parabolas	Parabolas	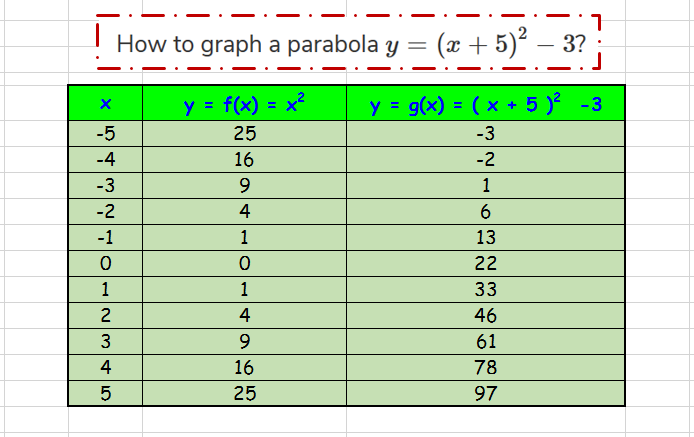Parabolas
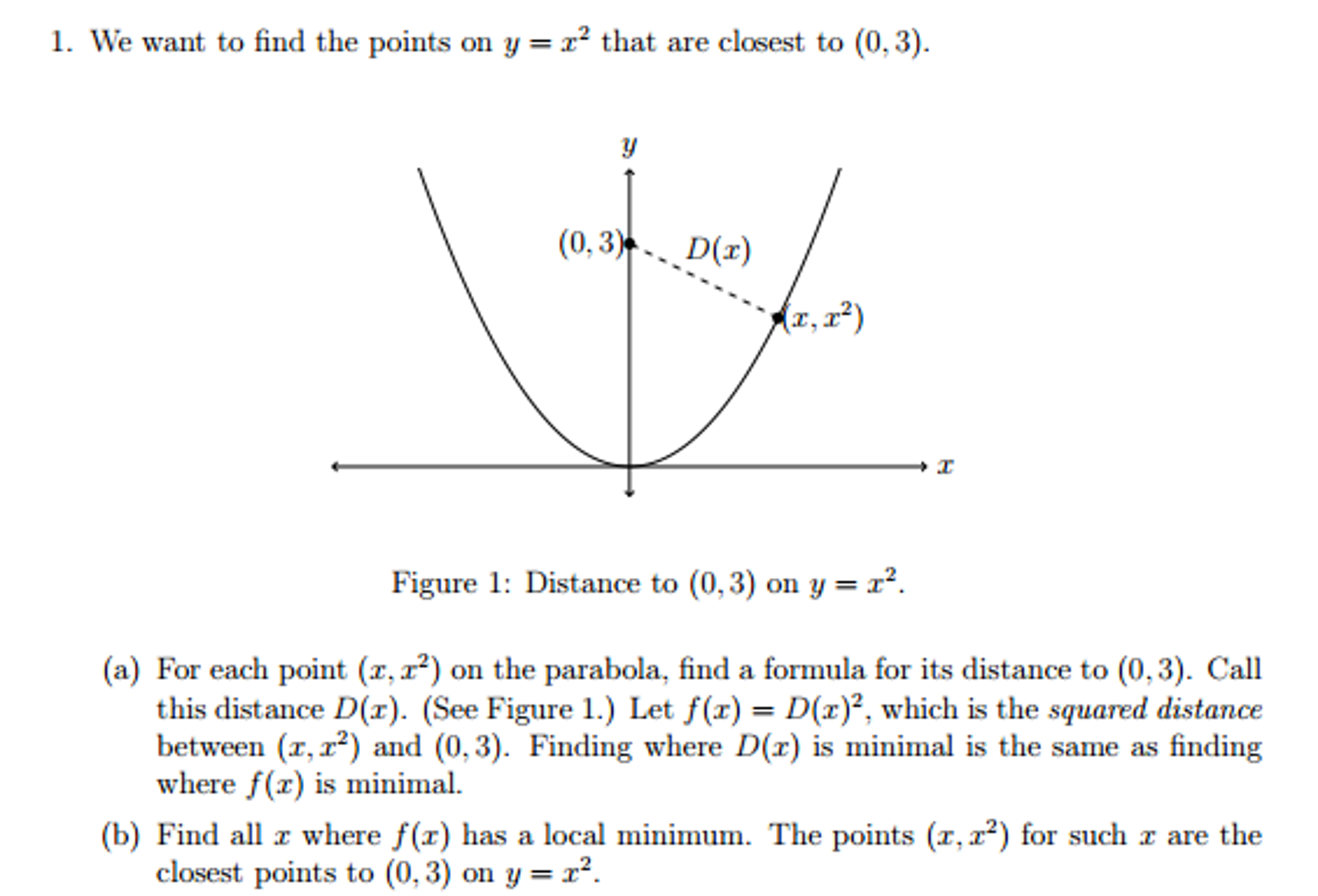Parabolas	Parabolas	Parabolas
Parabolas	Parabolas	Parabolas
Parabolas	Parabolas	Parabolas
Parabolas	Parabolas	Parabolas
Parabolas	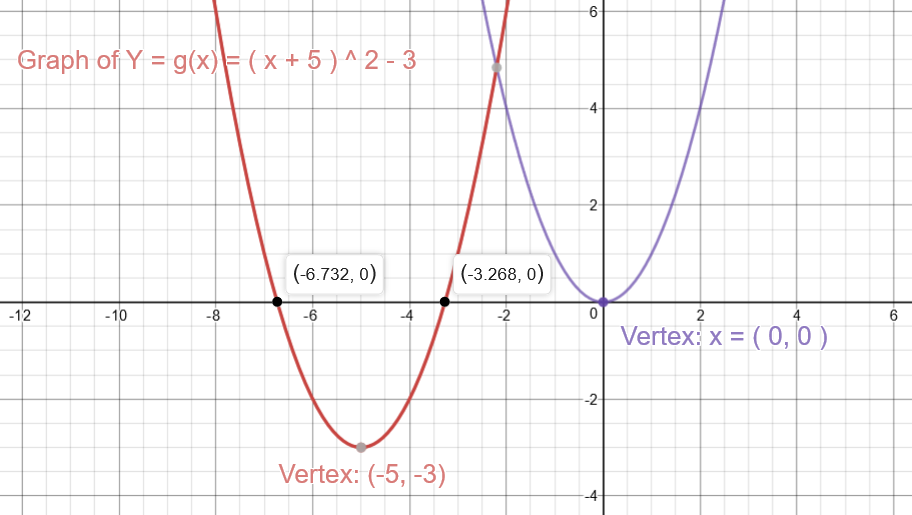Parabolas	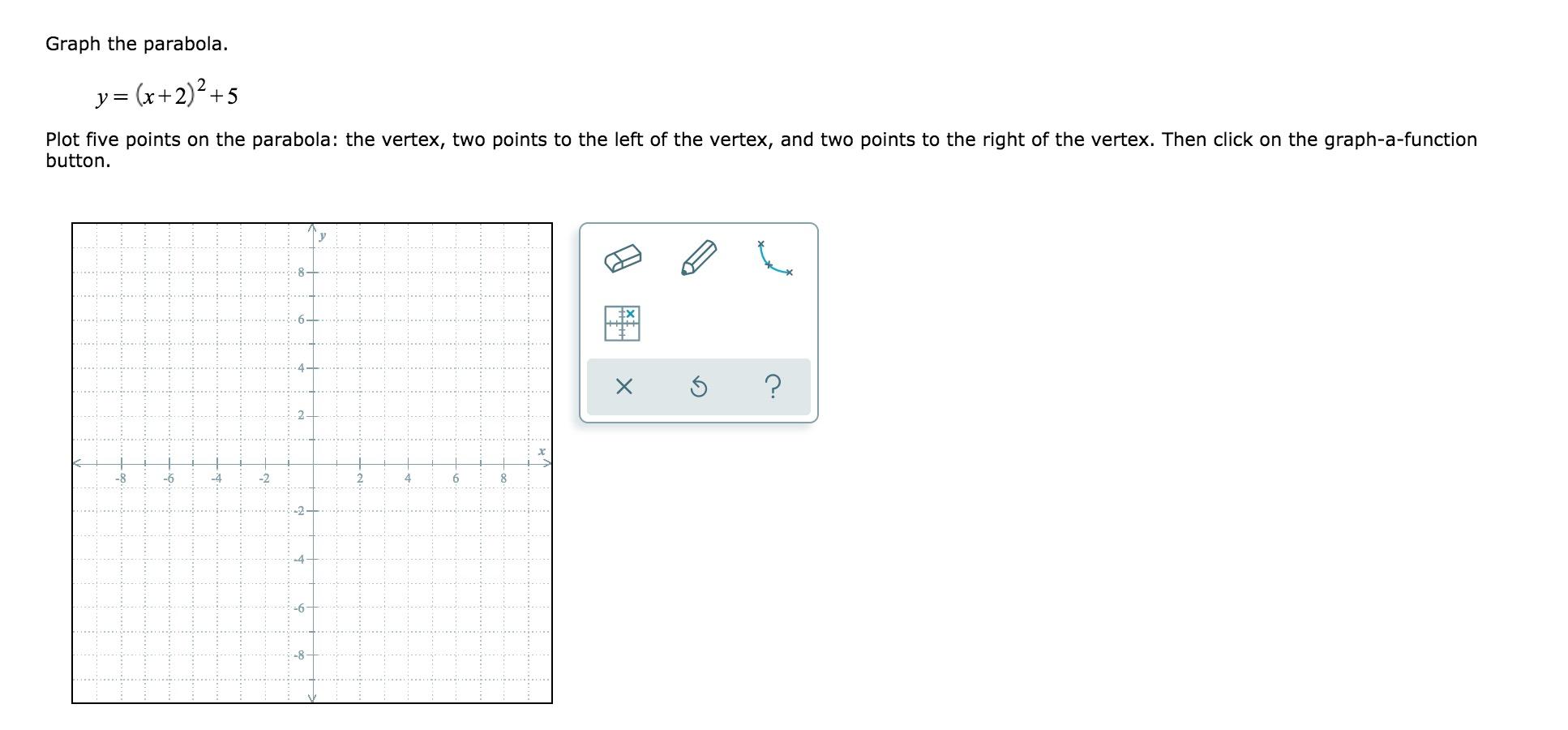Parabolas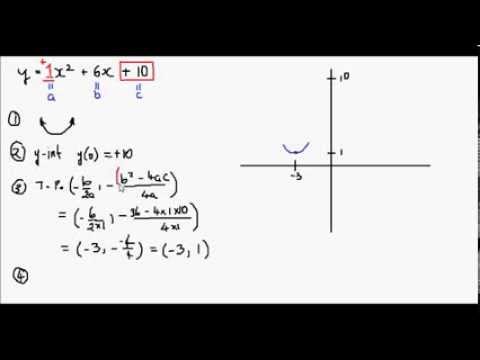
Parabolas	Parabolas	Parabolas
Parabolas	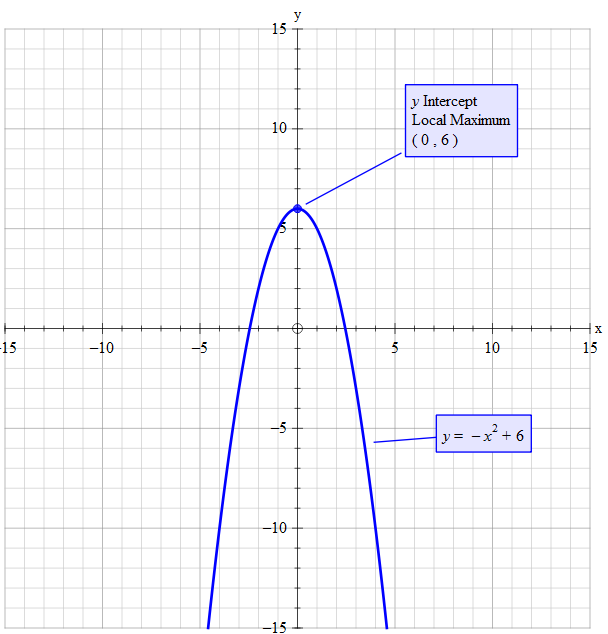Parabolas	Parabolas
Parabolas	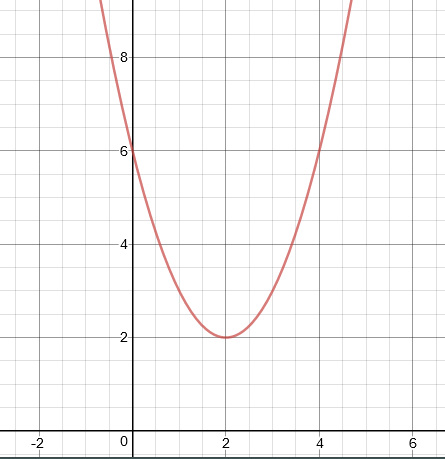Parabolas	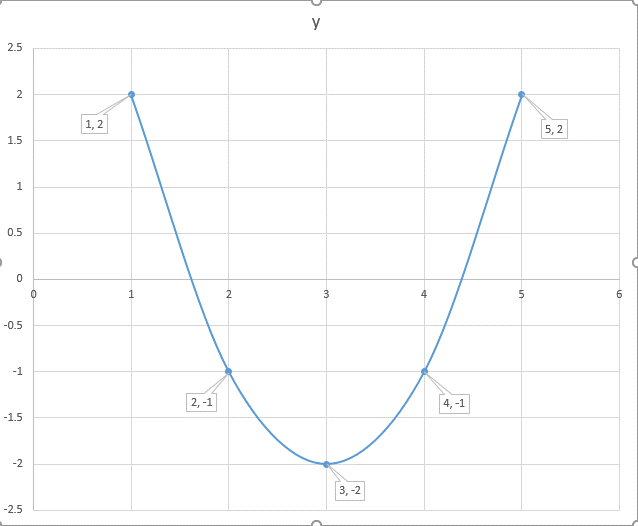Parabolas
Parabolas	Parabolas	Parabolas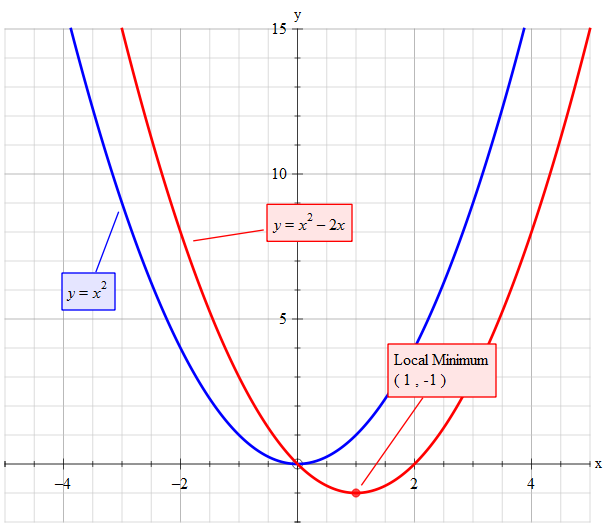
Parabolas	Parabolas	Parabolas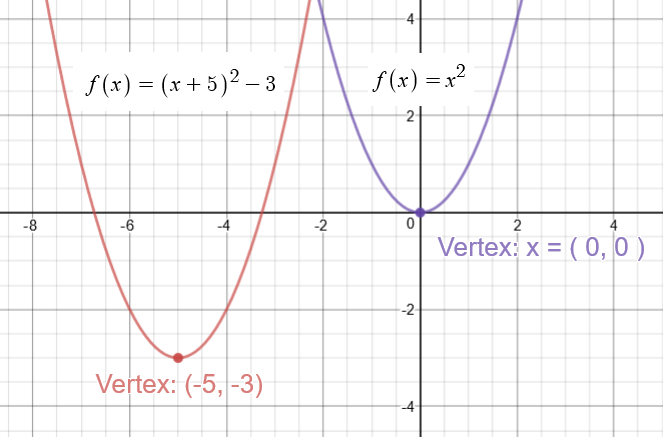
Parabolas	Parabolas	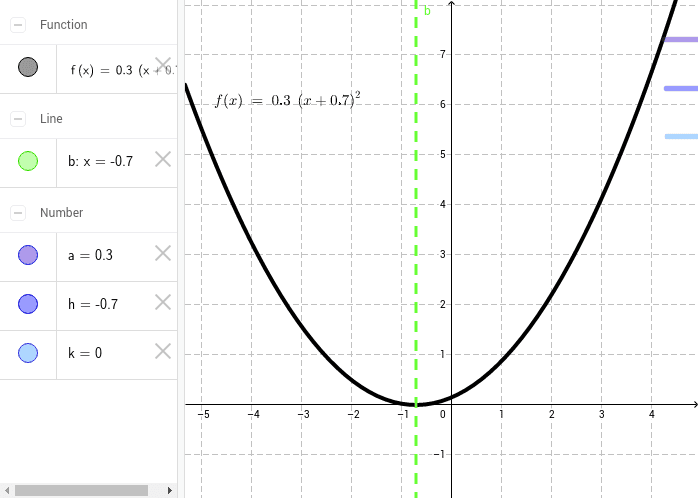Parabolas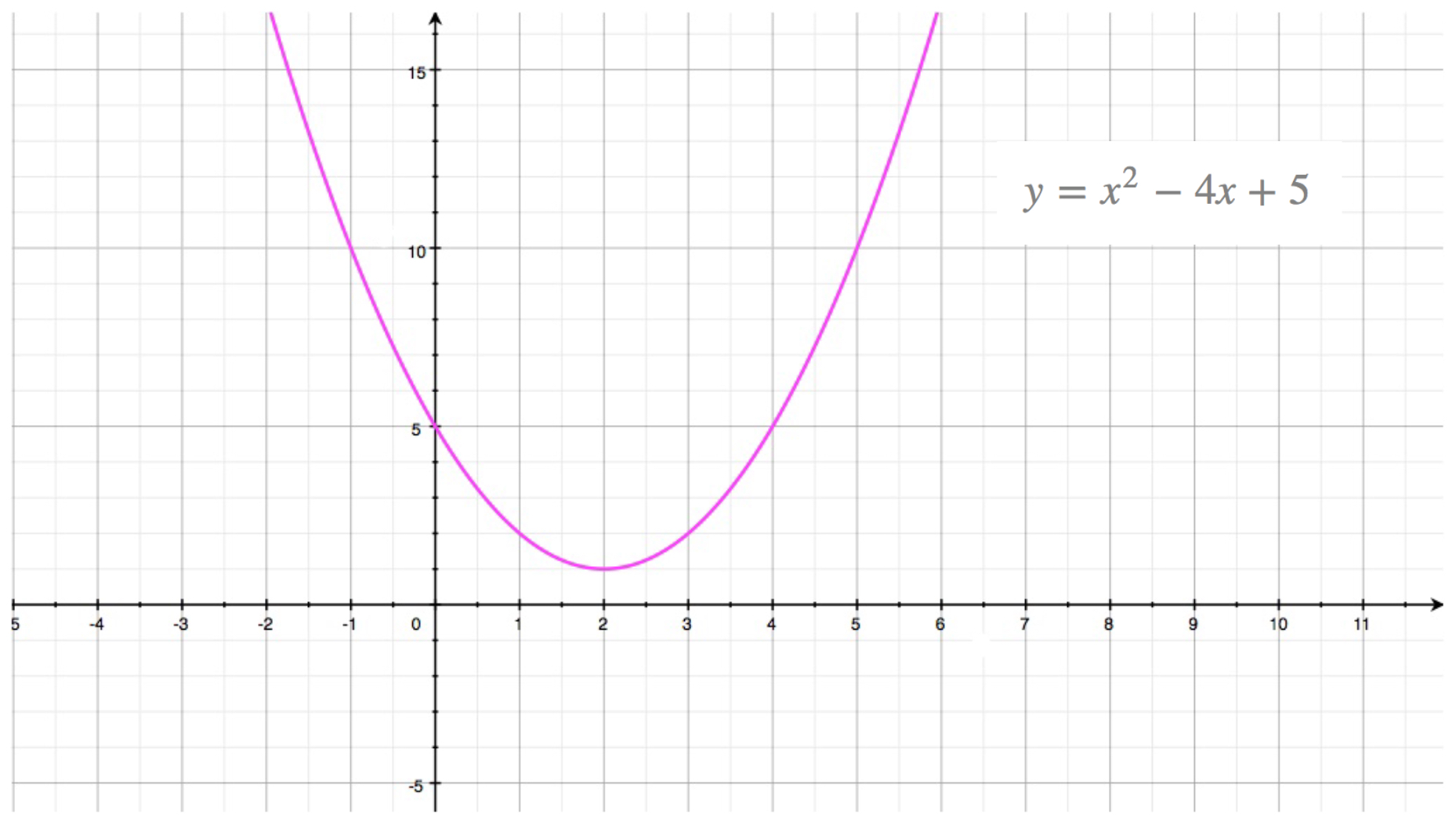
Parabolas	Parabolas	Parabolas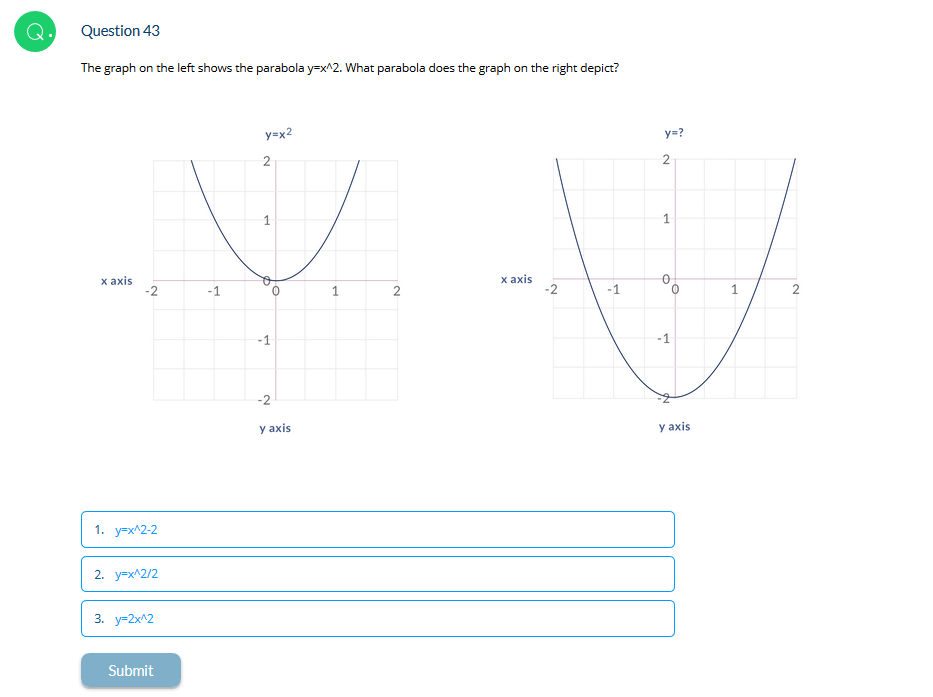
Parabolas	Parabolas	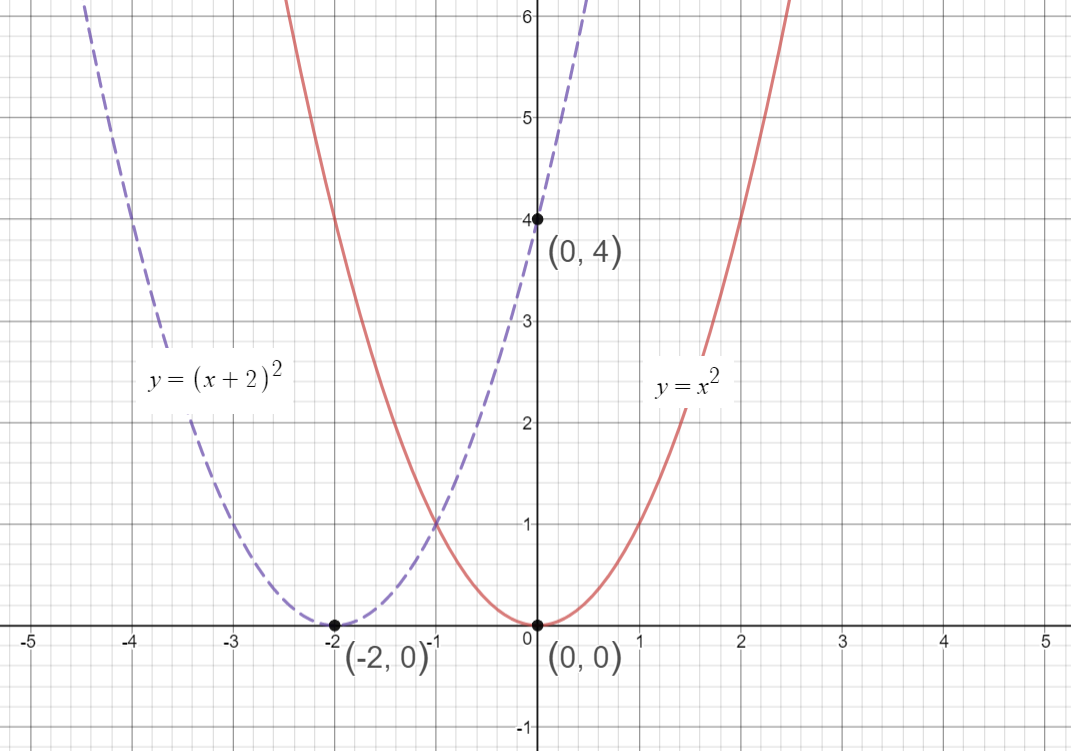Parabolas
Parabolas	Parabolas	Parabolas
Parabolas	Parabolas	Parabolas
Parabolas	Parabolas	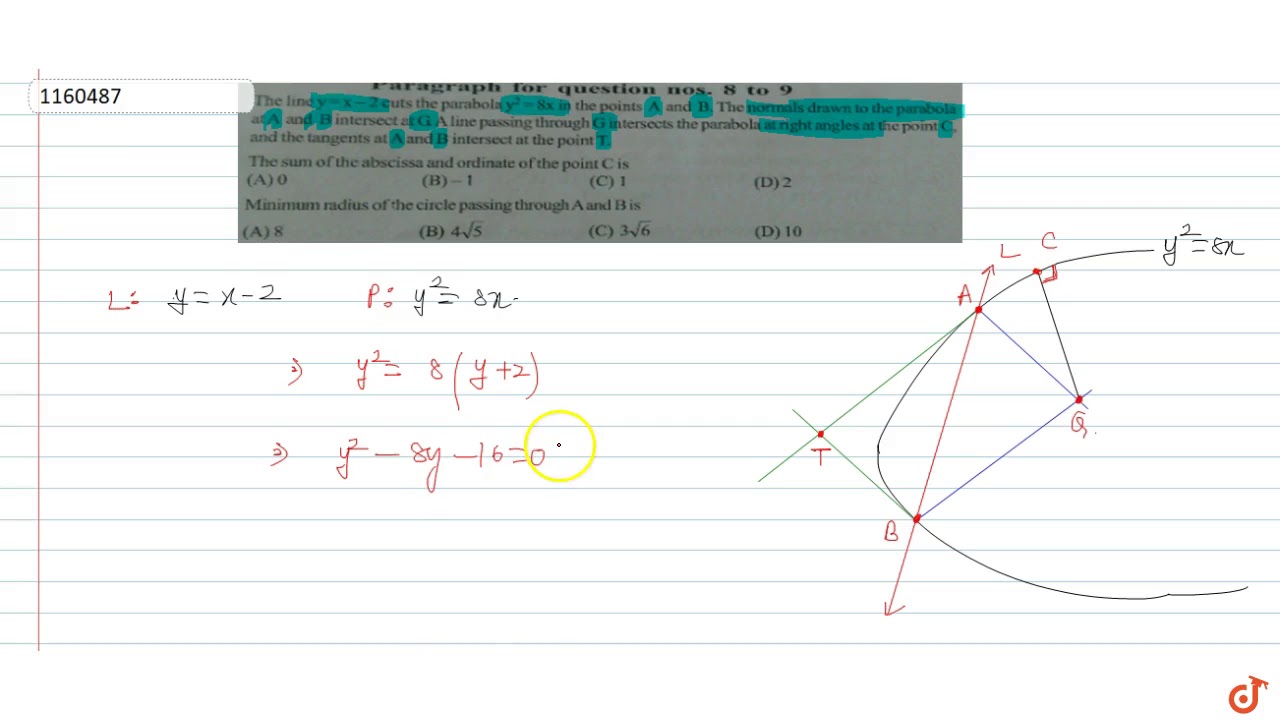Parabolas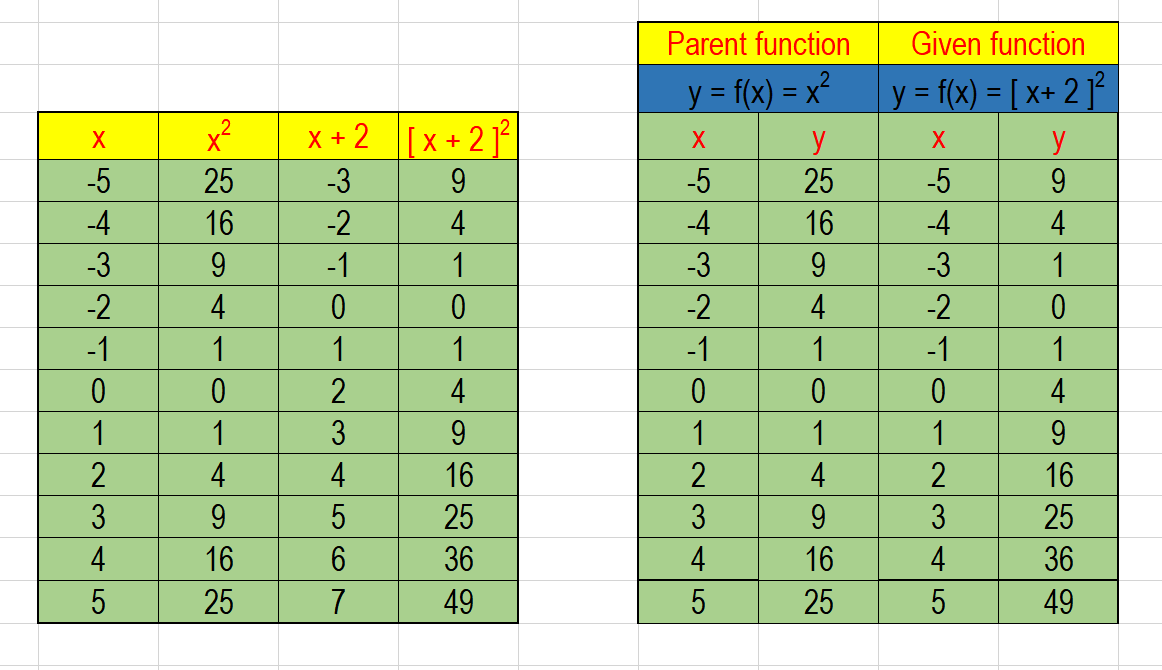
Parabolas	Parabolas	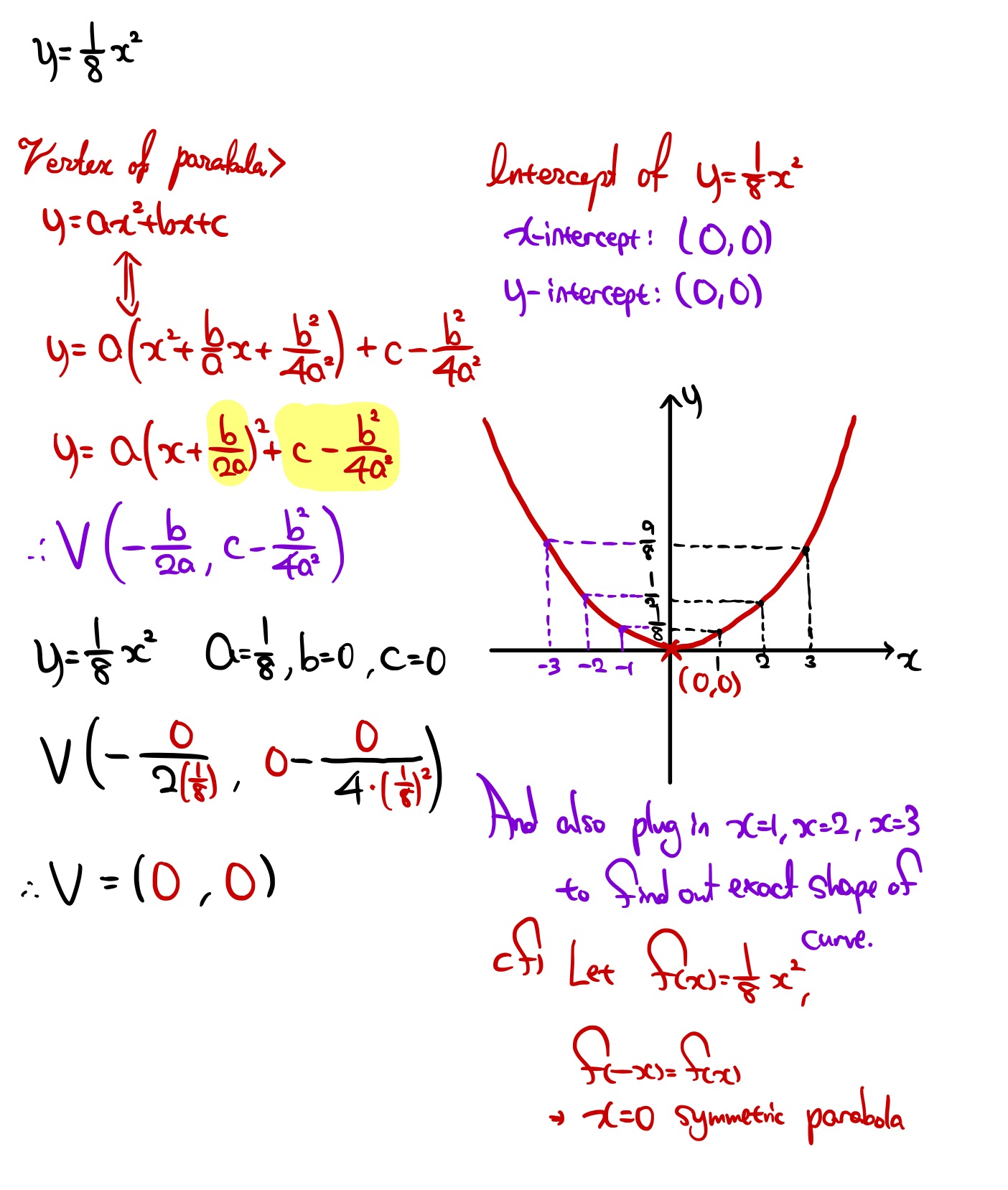Parabolas
Parabolas	Parabolas	Parabolas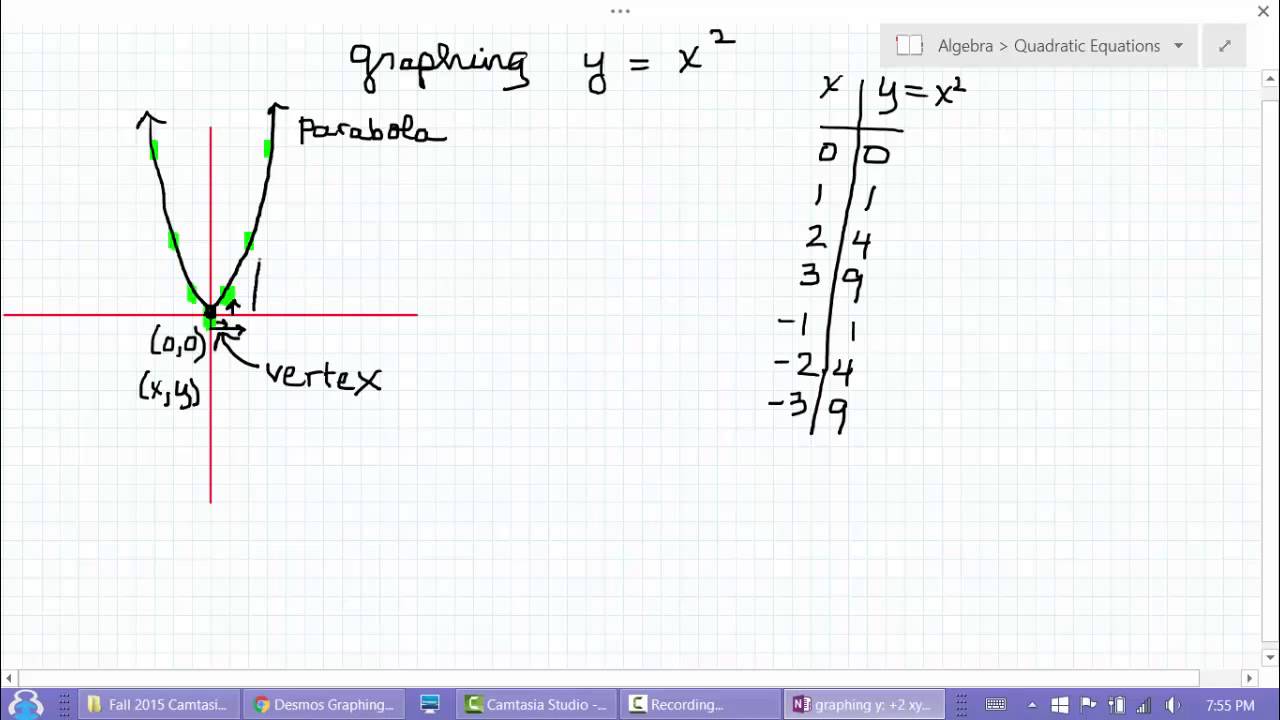
Parabolas	Parabolas	Parabolas
Parabolas	Parabolas	Parabolas
Parabolas	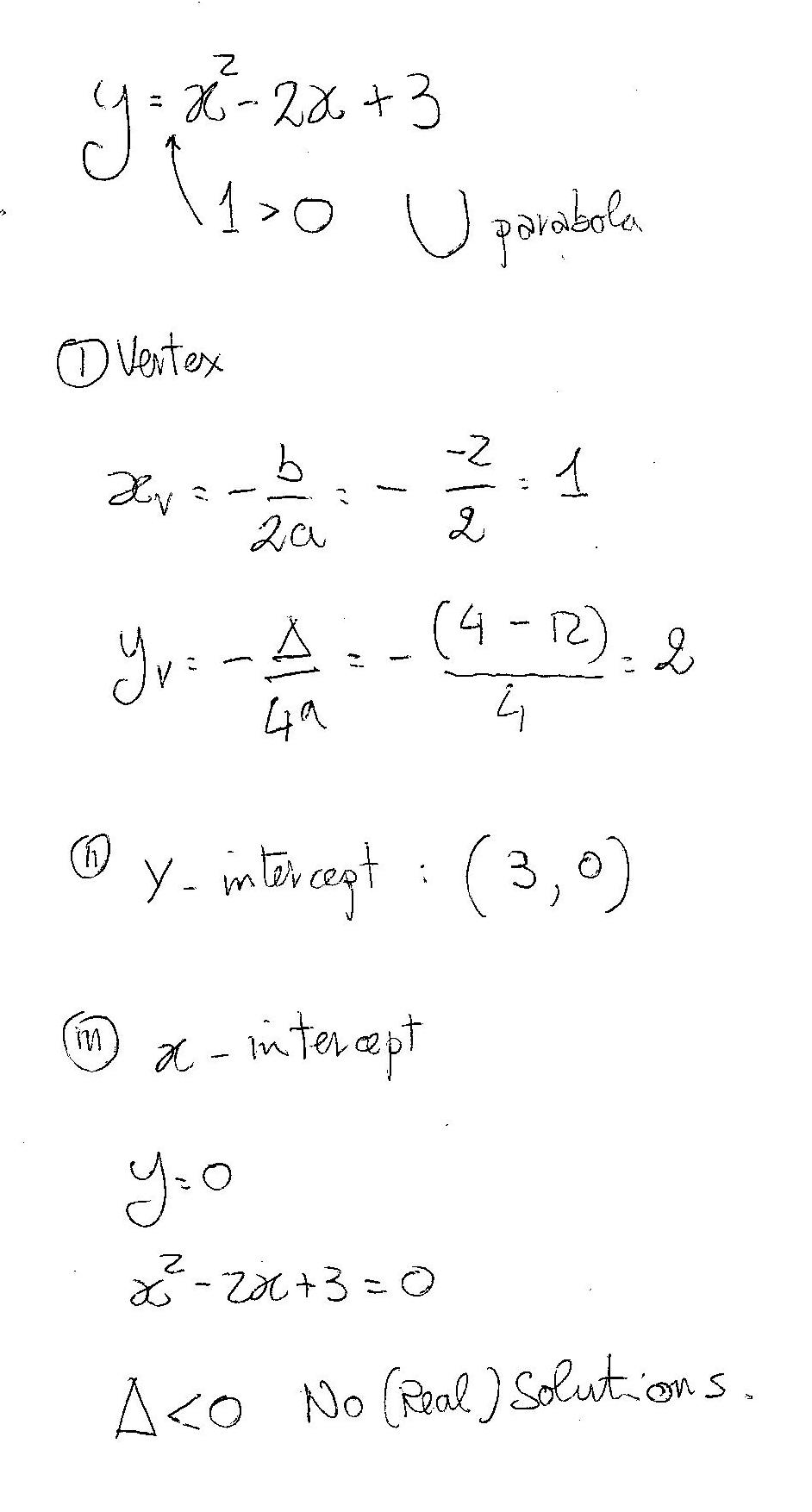Parabolas	Parabolas
Parabolas	Parabolas	Parabolas
Parabolas	Parabolas	Parabolas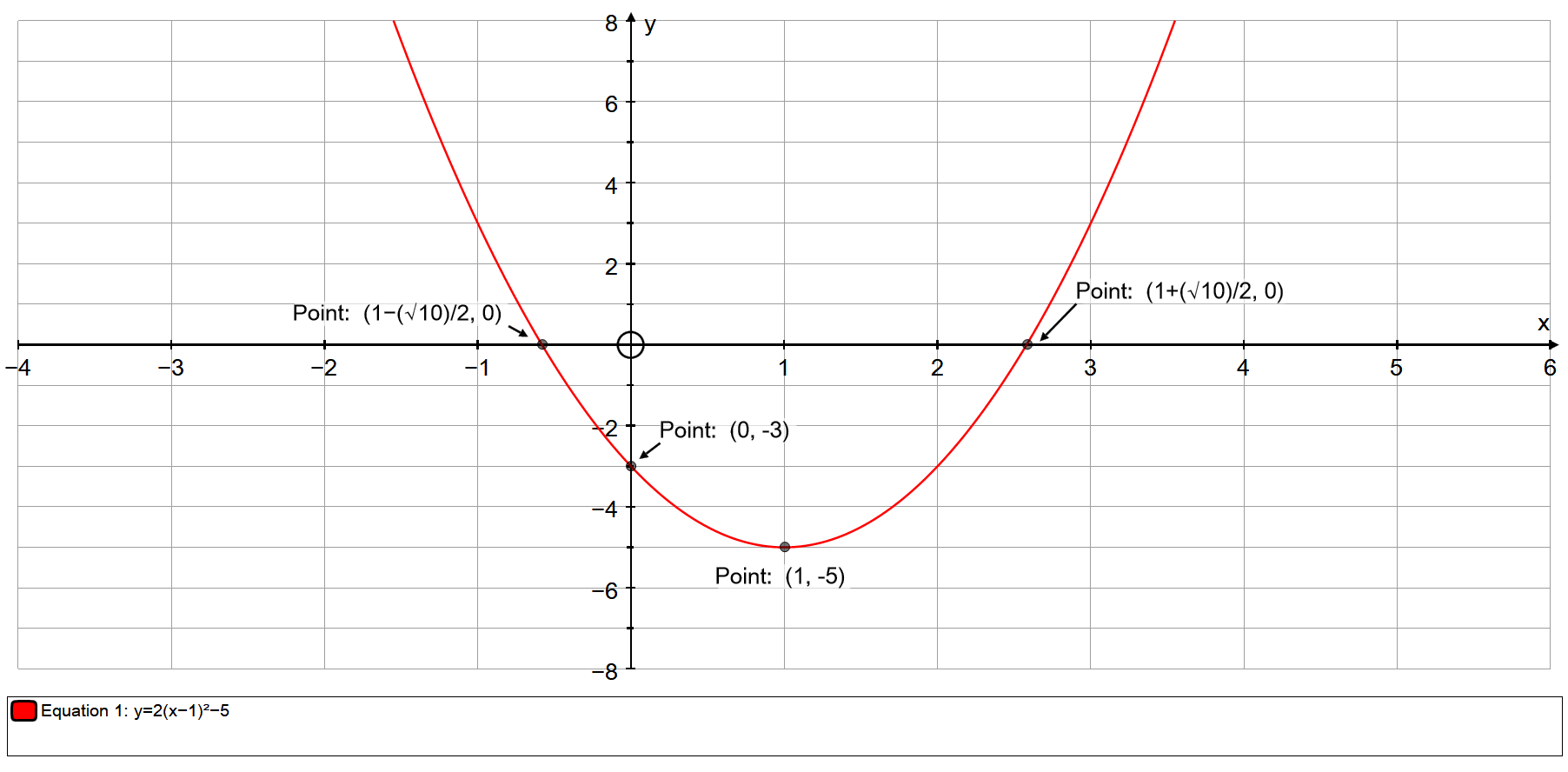
Parabolas	Parabolas	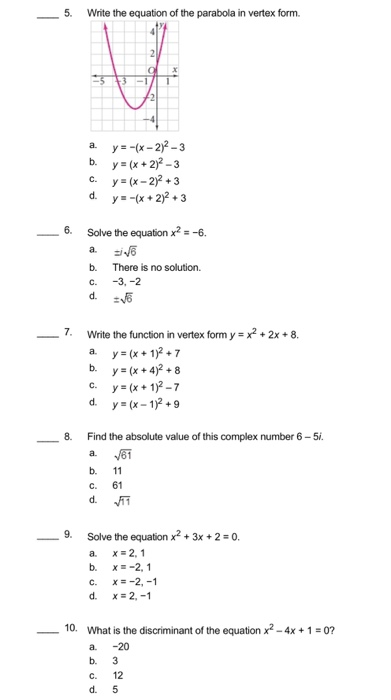Parabolas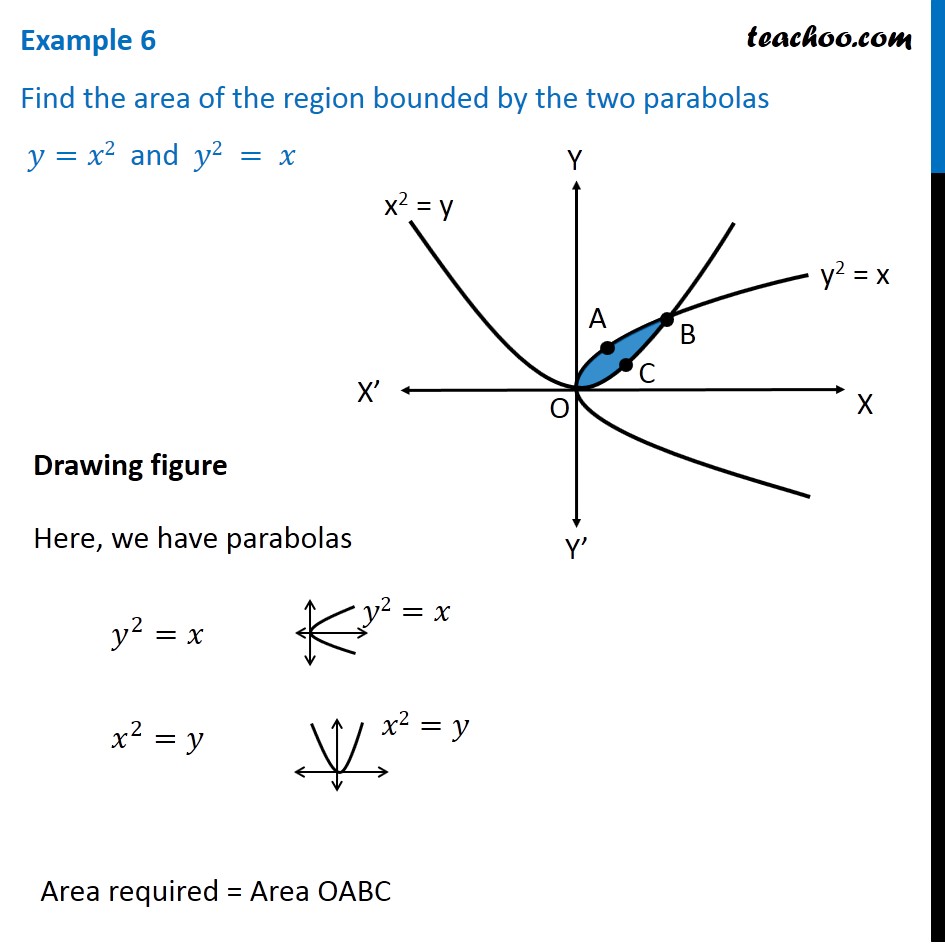
Parabolas	Parabolas	Parabolas
Parabolas	Parabolas	Parabolas
Parabolas	Parabolas	Parabolas
Parabolas	Parabolas	Parabolas
Parabolas	Parabolas	Parabolas
Parabolas	Parabolas	Parabolas
Parabolas	Parabolas	Parabolas
Parabolas	Parabolas	Parabolas
Parabolas	Parabolas	Parabolas
Parabolas	Parabolas

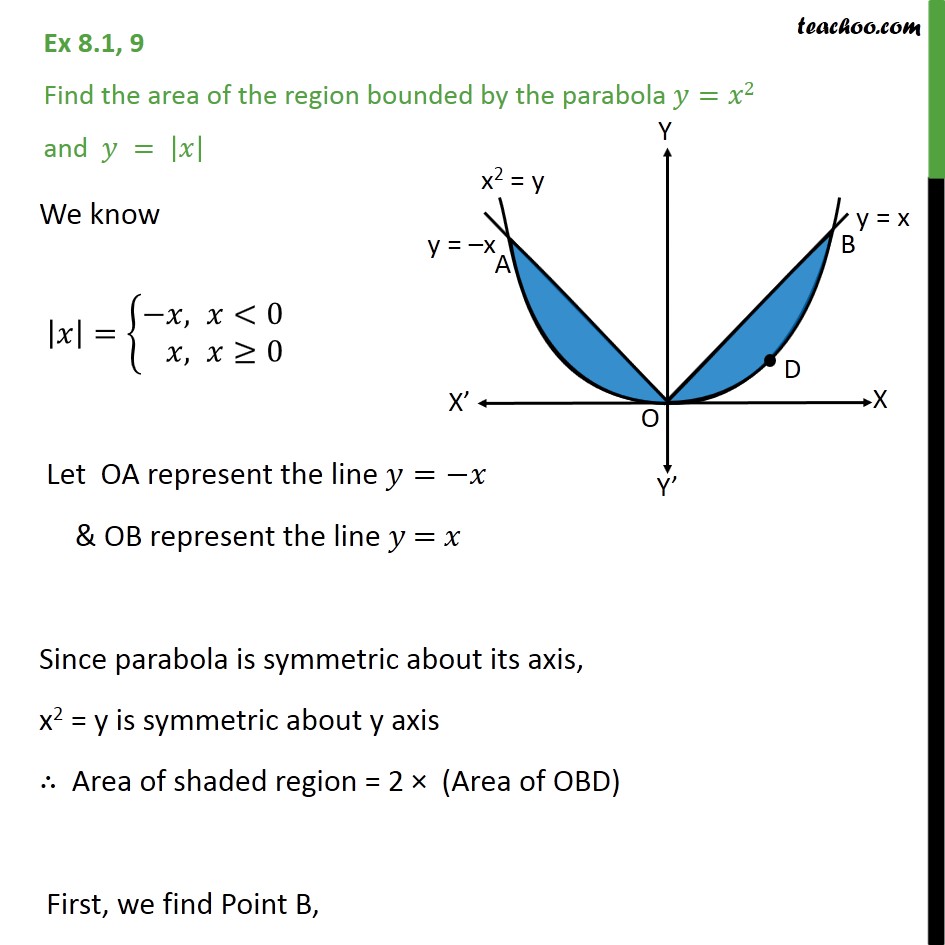
 answered The parabola y=x^2y=x 2 y, equals, x, squared is scaled vertically by a factor of \dfrac23 3 2 start fraction, 2, divided by, 3, end fraction What is the equation of theThe order of the differential equation of all tangent lines to the parabola y = x^2 is Question The order of the differential equation of all tangent lines to the parabola y = x 2 is A 1 Right on!

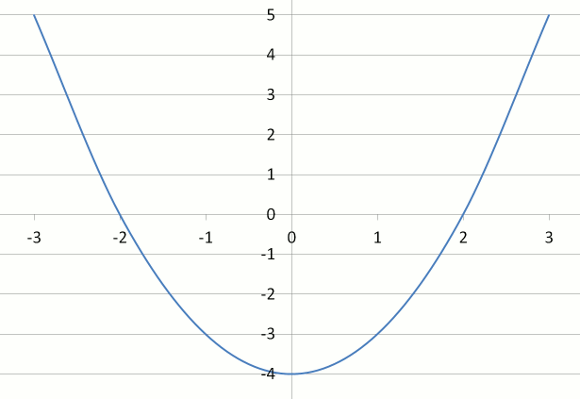
Incoming Term: y=x^2+2 parabola, y=(x-2)^2-3 parabola, (y+5/2)^2=-5(x-9/2) parabola, y=(x+4)^2-2 parabola, y=x^2-4x+2 parabola, y=x^2+3x+2 parabola, parabola y=x^2-2 direfleksikan, y=x^2 parabola graph, graph the parabola y=(x-2)^2-3, (x-2)2=8(y-1) parabola,